Aufgaben:Aufgabe 2.12Z: Reed–Solomon–Syndromberechnung: Unterschied zwischen den Versionen
Aus LNTwww
| Zeile 36: | Zeile 36: | ||
===Fragebogen=== | ===Fragebogen=== | ||
<quiz display=simple> | <quiz display=simple> | ||
| − | { | + | {Empfangen wurde $\underline{y} = (\alpha, \, 0, \, \alpha^3, \, 0, \, 1, \, \alpha, \, 0)$. Geben Sie das erste Element des Syndroms $\underline{s} = (s_0, \, s_1, \, s_2)$ an. |
|type="()"} | |type="()"} | ||
| − | + | + | + $s_0 = \alpha^4$, |
| − | - | + | - $s_0 = \alpha^5$, |
| + | - $s_0 = \alpha^6$, | ||
| + | - $s_0 = 0, \, 1, \, \alpha, \, \alpha^2$ oder $\alpha^3$. | ||
| − | { | + | {Wie lautet bei gleichem Empfangswort das zweite Syndromelement? |
|type="()"} | |type="()"} | ||
| − | + | + | - $s_1 = \alpha^4$, |
| − | - | + | + $s_1 = \alpha^5$, |
| + | - $s_1 = \alpha^6$, | ||
| + | - $s_1 = 0, \, 1, \, \alpha, \, \alpha^2$ oder $\alpha^3$. | ||
| − | { | + | {Wie lautet bei gleichem Empfangswort das dritte Syndromelement? |
|type="()"} | |type="()"} | ||
| − | + | + | - $s_2 = \alpha^4$, |
| − | - | + | - $s_2 = \alpha^5$, |
| + | + $s_2 = \alpha^6$, | ||
| + | - $s_2 = 0, \, 1, \, \alpha, \, \alpha^2$ oder $\alpha^3$. | ||
| − | { | + | {Bekannt ist, dass das vorliegende Empfangswort $\underline{y}$ decodiert werden kann. Wieviele Symbolfehler beinhaltet das Empfangswort? |
|type="{}"} | |type="{}"} | ||
| − | $ | + | $r \ = \ ${ 1 3% } |
</quiz> | </quiz> | ||
Version vom 18. Dezember 2017, 13:01 Uhr
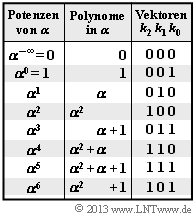
Wie in der Aufgabe A2.12 betrachten wir den Reed–Solomon–Code $(7, \, 4, \, 4)_8$, der auf dem Galoisfeld ${\rm GF}(q)$ mit $q = 8 = 2^3$ basiert. Die Grafik zeigt die zugehörige Umrechnungstabelle.
Gegeben sind die möglichen Codesymbole in Exponentendarstellung (Potenzen von $\alpha$) sowie in Polynom– und Koeffizientendarstellung.
Vorgegeben ist das Empfangswort $\underline{y} = (\alpha, \, 0, \, \alpha^3, \, 0, \, 1, \, \alpha, \, 0)$. Anhand des Syndroms
- $$\underline {s} = (s_0, s_1, s_2) = \underline {y} \cdot { \boldsymbol{\rm H }}^{\rm T}$$
soll überprüft werden, ob einzelne Symbole des Empfangsvektors $\underline{y}$ bei der Übertragung verfälscht wurden. Gegeben ist hierzu die Prüfmatrix $\mathbf{H}$ des betrachteten Codes und deren Transponierte:
- $${ \boldsymbol{\rm H}} = \begin{pmatrix} 1 & \alpha^1 & \alpha^2 & \alpha^3 & \alpha^4 & \alpha^5 & \alpha^6\\ 1 & \alpha^2 & \alpha^4 & \alpha^6 & \alpha^1 & \alpha^{3} & \alpha^{5}\\ 1 & \alpha^3 & \alpha^6 & \alpha^2 & \alpha^{5} & \alpha^{1} & \alpha^{4} \end{pmatrix} \hspace{0.05cm},\hspace{0.4cm} { \boldsymbol{\rm H}}^{\rm T} = \begin{pmatrix} 1 & 1 & 1 \\ \alpha^1 & \alpha^2 & \alpha^3 \\ \alpha^2 & \alpha^4 & \alpha^6 \\ \alpha^3 & \alpha^6 & \alpha^2 \\ \alpha^4 & \alpha^1 & \alpha^{5} \\ \alpha^5 & \alpha^{3} & \alpha^{1} \\ \alpha^6 & \alpha^{5} & \alpha^{4} \end{pmatrix} \hspace{0.05cm}.$$
Hinweise:
- Die Aufgabe bezieht auf die Seite 4 des Kapitels Fehlercodierung nach Reed–Solomon–Codierung.
- Sollte die Eingabe des Zahlenwertes „0” erforderlich sein, so geben Sie bitte „0.” ein.
Fragebogen
Musterlösung
(1)
(2)
(3)
(4)
(5)