Aufgaben:Aufgabe 1.11Z: Nochmals Syndromdecodierung

• Zurück zum Buch

Schaubild der Prüfgleichungen

Betrachtet wird die gleiche Konstellation wie in der Aufgabe 1.11, nämlich die Decodierung eines (7, 4, 3)–Hamming–Codes mit der Prüfmatrix

$${ \boldsymbol{\rm H}}_{\rm } = \begin{pmatrix} 1 &1 &0 &1 &1 &0 &0\\ 0 &1 &1 &1 &0 &1 &0\\ 1 &0 &1 &1 &0 &0 &1 \end{pmatrix} \hspace{0.05cm}.$$

Dementsprechend lautet das Generatorpolynom:

$${ \boldsymbol{\rm G}} = \begin{pmatrix} 1 &0 &0 &0 &1 &0 &1\\ 0 &1 &0 &0 &1 &1 &0\\ 0 &0 &1 &0 &0 &1 &1\\ 0 &0 &0 &1 &1 &1 &1 \end{pmatrix}\hspace{0.05cm}.$$

Bei der Syndromdecodierung bildet man aus dem Empfangsvektor y das Syndrom s:

$$\underline{s} = \underline{y} \cdot { \boldsymbol{\rm H}}^{\rm T} \in {\rm GF}(2^m) \hspace{0.05cm}.$$

Mit diesem Ergebnis lässt sich beim betrachteten Hamming–Code ein jeder Einzelfehler im Codewort korrigieren. Im fehlerfreien Fall gilt $\underline{s} = \underline{s}_{0} = (0, 0, 0)$. Aber auch bei 3 Übertragungsfehlern kann sich unter Umständen $\underline{s}_{0} = (0, 0, 0)$ ergeben, so dass diese Fehler unerkannt bleiben.

Hinweis:

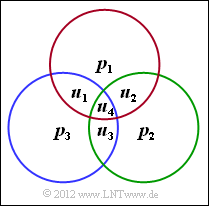
Die Aufgabe bezieht sich auf die im Kapitel Decodierung linearer Blockcodes behandelte Thematik. Weitere Informationen zur Syndromdecodierung finden Sie im Angabenblatt zur Aufgabe 1.11. Die Grafik verdeutlicht die drei Prüfgleichungen entsprechend der Prüfmatrix:

• erste Zeile: rote Gruppierung,
• zweite Zeile: grüne Gruppierung,
• dritte Zeile: blaue Gruppierung.

Fragebogen

1

{Handelt es sich um einen systematischen Code?

	Ja,
	Nein.

2

Empfangen wurde $\underline{y} = (1, 0, 0, 1, 0, 1, 0)$. Ist dies ein gültiges Codewort?

	Ja,
	Nein.

3

Welches Syndrom ergibt sich mit diesem Empfangswort?

	$\underline{s} = \underline{s}_{0} = (0, 0, 0),$
	$\underline{s} = \underline{s}_{3} = (0, 1, 1),$
	$\underline{s} = \underline{s}_{7} = (1, 1, 1).$

4

Welche Empfangsworte führen zum gleichen Syndrom wie in Teilaufgabe (3)?

	$\underline{y} = (1, 1, 0, 1, 0, 1, 0),$
	$\underline{y} = (0, 1, 0, 1, 0, 0, 1),$
	$\underline{y} = (0, 1, 1, 0, 1, 0, 1).$

Musterlösung

Musterlösung

(1)  Die Antwort ist JA, wie man aus der vorgegebenen Prüfmatrix H erkennt. Diese beinhaltet am Ende eine 3×3–Diagonalmatrix. Die Codeworte lauten demzufolge:

$$ \underline{x} = ( x_1, x_2, x_3, x_4, x_5, x_6, x_7) = ( u_1, u_2, u_3, u_4, p_1, p_2, p_{3}) \hspace{0.05cm}.$$

(2)  Mit diesem Empfangsvektor y werden alle Prüfgleichungen erfüllt:

$$u_1 \oplus u_2 \oplus u_4 \oplus p_1 = 1 \oplus 0 \oplus 1 \oplus 0 = 0 \hspace{0.05cm},\\ u_2 \oplus u_3 \oplus u_4 \oplus p_2 = 0 \oplus 0 \oplus 1 \oplus 1 = 0 \hspace{0.05cm},\\ u_1 \oplus u_3 \oplus u_4 \oplus p_3 = 1 \oplus 0 \oplus 1 \oplus 0 = 0 \hspace{0.05cm}.$$

Richtig ist dementsprechend die Antwort JA.

(3)  Es gilt $\underline{s} = \underline{y} · \boldsymbol{\rm H}^{\rm T}$:

$$ \underline{s} = \begin{pmatrix} 1 &0 &0 &1 &0 &1 &0 \end{pmatrix} \cdot \begin{pmatrix} 1 &0 &1\\ 1 &1 &0\\ 0 &1 &1\\ 1 &1 &1\\ 1 &0 &0\\ 0 &1 &0\\ 0 &0 &1 \end{pmatrix} = \begin{pmatrix} 0 &0 &0 \end{pmatrix} = \underline{s}_0 \hspace{0.2cm} \Rightarrow\hspace{0.2cm} \hspace{0.15cm} \underline{ \rm Antwort \hspace{0.15cm}1} \hspace{0.05cm}.$$

(4)  Man könnte nun für jedes y die Gleichung $\underline{y} · \underline{y} · \boldsymbol{\rm H}^{\rm T} = (0, 0, 0)$ überprüfen. Hier soll nun das Ergebnis auf anderem Wege gewonnen werden:

• $\underline{y}= (1, 1, 0, 1, 0, 1, 0)$ unterscheidet sich von $\underline{y} = (1, 0, 0, 1, 0, 1, 0)$ im Bit $u_{2}$, das nur in den beiden ersten Prüfgleichungen verwendet wird, nicht jedoch in der letzten ⇒ $\underline{s} = \underline{s}_{6} = (1, 1, 0)$.

• Wendet man die Prüfgleichungen auf $\underline{y} = (0, 1, 0, 1, 0, 0, 1)$ an, so erhält man $\underline{s} = \underline{s}_{0} = (0, 0, 0)$, wie die folgende Rechnung belegt:

$$u_1 \oplus u_2 \oplus u_4 \oplus p_1 = 0 \oplus 1 \oplus 1 \oplus 0 = 0 \hspace{0.05cm},\\ u_2 \oplus u_3 \oplus u_4 \oplus p_2 = 1 \oplus 0 \oplus 1 \oplus 0 = 0 \hspace{0.05cm},\\ u_1 \oplus u_3 \oplus u_4 \oplus p_3 = 0 \oplus 0 \oplus 1 \oplus 1 = 0 \hspace{0.05cm}.$$

• Zum gleichen Ergebnis kommt man mit dem Empfangsvektor $\underline{y} = (0, 1, 1, 0, 1, 0, 1),$ der sich vom Vektor (1, 0, 0, 1, 0, 1, 0) in allen 7 Bitpositionen unterscheidet:

$$u_1 \oplus u_2 \oplus u_4 \oplus p_1 = 0 \oplus 1 \oplus 0 \oplus 1 = 0 \hspace{0.05cm},\\ u_2 \oplus u_3 \oplus u_4 \oplus p_2 = 1 \oplus 1 \oplus 0 \oplus 0 = 0 \hspace{0.05cm},\\ u_1 \oplus u_3 \oplus u_4 \oplus p_3 = 0 \oplus 1 \oplus 0 \oplus 1 = 0 \hspace{0.05cm}.$$

Richtig sind also die Antworten 2 und 3.