Aufgabe 4.1Z: L–Werte des BEC–Modells
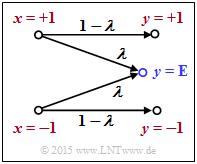
Wir betrachten das so genannte BEC–Kanalmodell (Binary Erasure Channel) mit
- der Eingangsgröße x∈{+1,−1},
- der Ausgangsgröße y∈{+1,−1,E}, und
- der Auslöschungswahrscheinlichkeit λ.
Hierbei bedeutet y=E (Erasure), dass der Ausgangswert y weder als +1 noch als −1 entschieden werden konnte.
Bekannt sind zudem die Eingangswahrscheinlichkeiten
- Pr(x=+1)=3/4,Pr(x=−1)=1/4.
Das Log–Likelihood–Verhältnis (kurz: L–Wert, englisch: Log Likelihood Ratio, LLR) der binären Zufallsgröße x ist bei bipolarer Betrachtungsweise wie folgt gegeben:
- L(x)=lnPr(x=+1)Pr(x=−1).
Entsprechend gilt für den bedingten L–Wert in Vorwärtsrichtung für alle y∈{+1,−1,E}:
- L(y|x)=lnPr(y|x=+1)Pr(y|x=−1).
Hinweise:
- Die Aufgabe gehört zum Kapitel Soft–in Soft–out Decoder.
- Bezug genommen wird insbesondere auf die Seite Zuverlässigkeitsinformation – Log Likelihood Ratio sowie auf die Seite Binary Erasure Channel.
Fragebogen
Musterlösung
- L(x)=lnPr(x=+1)Pr(x=−1)=ln3/41/4=1.099_.
(2) Entsprechend der Definition
- L(x)=lnPr(x=+1)Pr(x=−1)
ergibt sich für L(x)=−2 die folgende Bestimmungsgleichung:
- Pr(x=+1)1−Pr(x=+1)!=e−2≈0.135⇒1.135⋅Pr(x=+1)!=0.135⇒Pr(x=+1)=0.119,Pr(x=−1)=0.881_.
(3) Für den bedingten L–Wert L(y=E|x) in Vorwärtsrichtung gilt beim vorgegebenen BEC–Modell:
- L(y=E|x)=lnPr(y=E|x=+1)Pr(y=E|x=−1)=lnλλ=0_.
(4) Analog zur Musterlösung der Teilaufgabe (3) erhält man für y=±1:
- L(y=+1|x) = lnPr(y=+1|x=+1)Pr(y=+1|x=−1)=ln1−λ0⇒+∞_,
- L(y=−1|x) = lnPr(y=−1|x=+1)Pr(y=−1|x=−1)=ln01−λ⇒−∞_.
Richtig sind demnach die Lösungsvorschläge 1 und 2.
(5) Richtig ist der letzte Lösungsvorschlag:
- Für λ=0 (idealer Kanal) ergibt sich L(y=E|x)=ln(0/0) ⇒ unbestimmtes Ergebnis.
- Für λ=1 (vollständige Auslöschung, y≡E) sind L(y=+1|x) und L(y=−1|x) unbestimmt.