Aufgaben:Aufgabe 4.1Z: Übertragungsmaß: Unterschied zwischen den Versionen
| Zeile 83: | Zeile 83: | ||
===Musterlösung=== | ===Musterlösung=== | ||
{{ML-Kopf}} | {{ML-Kopf}} | ||
| − | '''(1)''' Setzt man in die gegebenen Gleichungen die Frequenz $f = 0$ ein, so erhält man | + | '''(1)''' Setzt man in die gegebenen Gleichungen die Frequenz $f = 0$ ein, so erhält man |
:$$\alpha(f = 0) = [1\,{\rm Np}] \hspace{-0.03cm}\cdot \hspace{-0.03cm} \sqrt{{1}/{2}\hspace{-0.03cm}\cdot \hspace{-0.03cm} R\hspace{0.03cm}' \hspace{-0.03cm}\cdot \hspace{-0.03cm} G\hspace{0.03cm}'+ {1}/{2}\cdot R\hspace{0.03cm}' \hspace{-0.03cm}\cdot \hspace{-0.03cm} | :$$\alpha(f = 0) = [1\,{\rm Np}] \hspace{-0.03cm}\cdot \hspace{-0.03cm} \sqrt{{1}/{2}\hspace{-0.03cm}\cdot \hspace{-0.03cm} R\hspace{0.03cm}' \hspace{-0.03cm}\cdot \hspace{-0.03cm} G\hspace{0.03cm}'+ {1}/{2}\cdot R\hspace{0.03cm}' \hspace{-0.03cm}\cdot \hspace{-0.03cm} | ||
G\hspace{0.03cm}'} = [1\,{\rm Np}] \hspace{-0.03cm}\cdot \hspace{-0.03cm} \sqrt{ R\hspace{0.03cm}' \hspace{-0.03cm}\cdot \hspace{-0.03cm} G\hspace{0.03cm}'} = [1\,{\rm Np}] \hspace{-0.03cm}\cdot \hspace{-0.03cm} \sqrt{ 100\,{\rm \Omega/km} \hspace{-0.03cm}\cdot \hspace{-0.03cm} 10^{-6}\,{\rm (\Omega \hspace{-0.03cm}\cdot \hspace{-0.03cm} km})^{-1}} | G\hspace{0.03cm}'} = [1\,{\rm Np}] \hspace{-0.03cm}\cdot \hspace{-0.03cm} \sqrt{ R\hspace{0.03cm}' \hspace{-0.03cm}\cdot \hspace{-0.03cm} G\hspace{0.03cm}'} = [1\,{\rm Np}] \hspace{-0.03cm}\cdot \hspace{-0.03cm} \sqrt{ 100\,{\rm \Omega/km} \hspace{-0.03cm}\cdot \hspace{-0.03cm} 10^{-6}\,{\rm (\Omega \hspace{-0.03cm}\cdot \hspace{-0.03cm} km})^{-1}} | ||
| Zeile 99: | Zeile 99: | ||
| − | '''(2)''' Mit $f = 10^{5} \ \rm Hz$ und den angegebenen Werten gilt | + | '''(2)''' Mit $f = 10^{5} \ \rm Hz$ und den angegebenen Werten gilt |
:$$f \cdot 2\pi L' = 10^5\,\frac{1}{ {\rm s}} \cdot 2 \cdot | :$$f \cdot 2\pi L' = 10^5\,\frac{1}{ {\rm s}} \cdot 2 \cdot | ||
10^{-3}\,\frac{\rm \Omega \cdot s}{ {\rm km}}= 200 \,\frac{\rm | 10^{-3}\,\frac{\rm \Omega \cdot s}{ {\rm km}}= 200 \,\frac{\rm | ||
| Zeile 117: | Zeile 117: | ||
| − | '''(3)''' Der Grenzübergang | + | '''(3)''' Der Grenzübergang für $f → \infty$ ergibt sich, wenn man im Zähler $R\hspace{0.03cm}'$ und im Nenner $G\hspace{0.08cm}'$ gegenüber den jeweils zweiten Term vernachlässigt: |
:$$\lim_{f \rightarrow \infty} \hspace{0.1cm} Z_{\rm W}(f) | :$$\lim_{f \rightarrow \infty} \hspace{0.1cm} Z_{\rm W}(f) | ||
= \lim_{\omega \rightarrow \infty} \hspace{0.1cm} \sqrt{\frac {R\hspace{0.03cm}' + {\rm j} \cdot \omega L'}{G' + {\rm j} \cdot \omega C\hspace{0.03cm}'}} | = \lim_{\omega \rightarrow \infty} \hspace{0.1cm} \sqrt{\frac {R\hspace{0.03cm}' + {\rm j} \cdot \omega L'}{G' + {\rm j} \cdot \omega C\hspace{0.03cm}'}} | ||
=\sqrt{\frac {2 \pi L\hspace{0.03cm}' }{2 \pi C\hspace{0.03cm}'}}=\sqrt{\frac {2 \cdot 10^{-3}\,{\rm \Omega \cdot s} } | =\sqrt{\frac {2 \pi L\hspace{0.03cm}' }{2 \pi C\hspace{0.03cm}'}}=\sqrt{\frac {2 \cdot 10^{-3}\,{\rm \Omega \cdot s} } | ||
{2 \cdot 10^{-73}\,{\rm s/\Omega} }} \hspace{0.15cm}\underline{= 100\,{\rm \Omega }}\hspace{0.05cm}.$$ | {2 \cdot 10^{-73}\,{\rm s/\Omega} }} \hspace{0.15cm}\underline{= 100\,{\rm \Omega }}\hspace{0.05cm}.$$ | ||
| − | Die Näherung für die Dämpfungsfunktion ist schwieriger herzuleiten. Ausgehend von | + | *Die Näherung für die Dämpfungsfunktion ist schwieriger herzuleiten. Ausgehend von |
:$$\alpha(\omega) = \sqrt{ {1}/{2}\cdot \left (R\hspace{0.03cm}' G\hspace{0.03cm}' - \omega^2 \cdot L\hspace{0.03cm}' C\hspace{0.03cm}'\right)+ | :$$\alpha(\omega) = \sqrt{ {1}/{2}\cdot \left (R\hspace{0.03cm}' G\hspace{0.03cm}' - \omega^2 \cdot L\hspace{0.03cm}' C\hspace{0.03cm}'\right)+ | ||
{1}/{2}\sqrt{(R\hspace{0.03cm}'\hspace{0.03cm}^2 + \omega^2 \cdot L\hspace{0.03cm}'\hspace{0.03cm}^2) \cdot (G\hspace{0.03cm}'\hspace{0.03cm}^2 + \omega^2 \cdot C\hspace{0.03cm}\hspace{0.03cm}'^2)}}$$ | {1}/{2}\sqrt{(R\hspace{0.03cm}'\hspace{0.03cm}^2 + \omega^2 \cdot L\hspace{0.03cm}'\hspace{0.03cm}^2) \cdot (G\hspace{0.03cm}'\hspace{0.03cm}^2 + \omega^2 \cdot C\hspace{0.03cm}\hspace{0.03cm}'^2)}}$$ | ||
| − | gilt dann ebenfalls: | + | :gilt dann ebenfalls: |
| − | :$$2 \cdot \alpha^2(\omega) = R' G' + \omega^2 \cdot L' | + | :$$2 \cdot \alpha^2(\omega) = R\hspace{0.03cm}' G\hspace{0.03cm}' + \omega^2 \cdot L' |
C'\cdot | C'\cdot | ||
| − | \left [-1 +\sqrt{(1 + \frac{R'^2}{ \omega^2 \cdot L'^2}) \cdot (1 + \frac{G'^2}{ \omega^2 \cdot C'^2})} \hspace{0.1cm} | + | \left [-1 +\sqrt{(1 + \frac{R\hspace{0.03cm}'^2}{ \omega^2 \cdot L\hspace{0.03cm}'^2}) \cdot (1 + \frac{G\hspace{0.03cm}'^2}{ \omega^2 \cdot C\hspace{0.03cm}'^2})} \hspace{0.1cm} |
\right]$$ | \right]$$ | ||
| − | :$$\Rightarrow \; \; 2 \cdot \alpha^2(\omega) \approx R' G' + \omega^2 \cdot L' | + | :$$\Rightarrow \; \; 2 \cdot \alpha^2(\omega) \approx R\hspace{0.03cm}' G\hspace{0.03cm}' + \omega^2 \cdot L' |
| − | C'\cdot | + | C\hspace{0.03cm}'\cdot |
| − | \left [-1 +\sqrt{1 + \frac{R'^2}{ \omega^2 \cdot L'^2}+ \frac{G'^2}{ \omega^2 \cdot C'^2}} \hspace{0.1cm} | + | \left [-1 +\sqrt{1 + \frac{R'^2}{ \omega^2 \cdot L'^2}+ \frac{G\hspace{0.03cm}'^2}{ \omega^2 \cdot C\hspace{0.03cm}'^2}} \hspace{0.1cm} |
\right].$$ | \right].$$ | ||
| − | Über die für kleine $x$ gültige Näherung $\sqrt{1 + x}\approx 1+x/2$ kommt man zum Zwischenergebnis für (unendlich) große Frequenzen: | + | *Über die für kleine $x$ gültige Näherung $\sqrt{1 + x}\approx 1+x/2$ kommt man zum Zwischenergebnis für (unendlich) große Frequenzen: |
| − | :$$2 \cdot \alpha^2(\omega \rightarrow \infty) = R' G' + \omega^2 \cdot L' | + | :$$2 \cdot \alpha^2(\omega \rightarrow \infty) = R\hspace{0.03cm}' G\hspace{0.05cm}' + \omega^2 \cdot L' |
| − | C'\cdot | + | C\hspace{0.05cm}'\cdot |
\left [ -1 +1 + {1}/{2} \cdot \left ( \frac{R\hspace{0.03cm}'\hspace{0.03cm}^2}{ \omega^2 \cdot L\hspace{0.03cm}'\hspace{0.03cm}^2}+ \frac{G\hspace{0.03cm}'\hspace{0.03cm}^2}{ \omega^2 \cdot C\hspace{0.03cm}'\hspace{0.03cm}^2} | \left [ -1 +1 + {1}/{2} \cdot \left ( \frac{R\hspace{0.03cm}'\hspace{0.03cm}^2}{ \omega^2 \cdot L\hspace{0.03cm}'\hspace{0.03cm}^2}+ \frac{G\hspace{0.03cm}'\hspace{0.03cm}^2}{ \omega^2 \cdot C\hspace{0.03cm}'\hspace{0.03cm}^2} | ||
\right) \hspace{0.1cm} | \right) \hspace{0.1cm} | ||
| Zeile 146: | Zeile 146: | ||
{1}/{2}\cdot \frac{R\hspace{0.03cm}' C\hspace{0.03cm}' + G\hspace{0.03cm}' L\hspace{0.03cm}'}{\sqrt{ C\hspace{0.03cm}' L\hspace{0.03cm}' }}= | {1}/{2}\cdot \frac{R\hspace{0.03cm}' C\hspace{0.03cm}' + G\hspace{0.03cm}' L\hspace{0.03cm}'}{\sqrt{ C\hspace{0.03cm}' L\hspace{0.03cm}' }}= | ||
{1}/{2}\cdot \left [R\hspace{0.03cm}' \cdot \sqrt{\frac{C\hspace{0.03cm}'}{L\hspace{0.03cm}'}}+G\hspace{0.03cm}' \cdot \sqrt{\frac{L\hspace{0.03cm}'}{C\hspace{0.03cm}'}}\right]\hspace{0.05cm}.$$ | {1}/{2}\cdot \left [R\hspace{0.03cm}' \cdot \sqrt{\frac{C\hspace{0.03cm}'}{L\hspace{0.03cm}'}}+G\hspace{0.03cm}' \cdot \sqrt{\frac{L\hspace{0.03cm}'}{C\hspace{0.03cm}'}}\right]\hspace{0.05cm}.$$ | ||
| − | Mit den eingesetzten Zahlenwerten ergibt sich | + | *Mit den eingesetzten Zahlenwerten ergibt sich |
:$$\alpha(f \rightarrow \infty) = \alpha(\omega \rightarrow \infty) | :$$\alpha(f \rightarrow \infty) = \alpha(\omega \rightarrow \infty) | ||
= {0.5\,{\rm Np/km}}\cdot \left [100 \cdot \sqrt{\frac{2 \cdot 10^{-7}}{2 \cdot10^{-3}}}+10^{-6} \cdot | = {0.5\,{\rm Np/km}}\cdot \left [100 \cdot \sqrt{\frac{2 \cdot 10^{-7}}{2 \cdot10^{-3}}}+10^{-6} \cdot | ||
| Zeile 154: | Zeile 154: | ||
| − | '''(4)''' Für kleine Frequenzen gilt $\omega L\hspace{0.03cm}' \ll R\hspace{0.03cm}'$ und $ \omega C\hspace{0.03cm}' \gg G\hspace{0.03cm}'$. Unter Vernachlässigung des $\omega^2$–Anteils erhält man: | + | '''(4)''' Für kleine Frequenzen gilt $\omega L\hspace{0.03cm}' \ll R\hspace{0.03cm}'$ und $ \omega C\hspace{0.03cm}' \gg G\hspace{0.03cm}'$. |
| + | *Unter Vernachlässigung des $\omega^2$–Anteils erhält man: | ||
:$$\alpha(f) = \sqrt{\frac {1}{2}\cdot \left (R\hspace{0.03cm}' G\hspace{0.03cm}' - \omega^2 \cdot L\hspace{0.03cm}' C\hspace{0.03cm}'\right)+ | :$$\alpha(f) = \sqrt{\frac {1}{2}\cdot \left (R\hspace{0.03cm}' G\hspace{0.03cm}' - \omega^2 \cdot L\hspace{0.03cm}' C\hspace{0.03cm}'\right)+ | ||
\frac {1}{2}\sqrt{(R\hspace{0.03cm}'\hspace{0.03cm}^2 + \omega^2 \cdot L\hspace{0.03cm}'\hspace{0.03cm}^2) \cdot (G\hspace{0.03cm}'\hspace{0.03cm}^2+ \omega^2 \cdot C\hspace{0.03cm}'\hspace{0.03cm}^2)}} | \frac {1}{2}\sqrt{(R\hspace{0.03cm}'\hspace{0.03cm}^2 + \omega^2 \cdot L\hspace{0.03cm}'\hspace{0.03cm}^2) \cdot (G\hspace{0.03cm}'\hspace{0.03cm}^2+ \omega^2 \cdot C\hspace{0.03cm}'\hspace{0.03cm}^2)}} | ||
| Zeile 165: | Zeile 166: | ||
{1}/{2} \cdot f \cdot R\hspace{0.03cm}' \cdot 2 \pi C\hspace{0.03cm}'} | {1}/{2} \cdot f \cdot R\hspace{0.03cm}' \cdot 2 \pi C\hspace{0.03cm}'} | ||
\hspace{0.05cm}.$$ | \hspace{0.05cm}.$$ | ||
| − | Hierbei ist berücksichtigt, dass der erste Anteil gemäß Teilaufgabe '''(1)''' außer bei der Frequenz $f = 0$ | + | *Hierbei ist berücksichtigt, dass der erste Anteil gemäß Teilaufgabe '''(1)''' außer bei der Frequenz $f = 0$ vernachlässigt werden kann. |
| − | *Für die Frequenz $f = 1 \ \rm kHz$ ergibt sich die Näherung | + | *Für die Frequenz $f = 1 \ \rm kHz$ ergibt sich die Näherung |
:$$\alpha(f = 1\,{\rm kHz}) = \sqrt{ | :$$\alpha(f = 1\,{\rm kHz}) = \sqrt{ | ||
{1}/{2} \cdot 10^{3}\,{\rm Hz} \cdot 100\,\frac{\rm \Omega }{ {\rm km}} \cdot 2 \cdot 10^{-7} | {1}/{2} \cdot 10^{3}\,{\rm Hz} \cdot 100\,\frac{\rm \Omega }{ {\rm km}} \cdot 2 \cdot 10^{-7} | ||
| Zeile 172: | Zeile 173: | ||
\hspace{0.15cm}\underline{= 0.1\,{\rm Np }/{ {\rm km}}} | \hspace{0.15cm}\underline{= 0.1\,{\rm Np }/{ {\rm km}}} | ||
\hspace{0.05cm}.$$ | \hspace{0.05cm}.$$ | ||
| − | *Für die Frequenz $f = | + | *Für die Frequenz $f = 4 \ \rm kHz$ ist das Dämpfungsmaß doppelt so groß: |
:$$\alpha(f = 4\,{\rm kHz}) \hspace{0.15cm}\underline{ = 0.2\,{\rm Np }/{ {\rm km}}} | :$$\alpha(f = 4\,{\rm kHz}) \hspace{0.15cm}\underline{ = 0.2\,{\rm Np }/{ {\rm km}}} | ||
\hspace{0.05cm}.$$ | \hspace{0.05cm}.$$ | ||
| Zeile 183: | Zeile 184: | ||
C\hspace{0.03cm}'}}= (1 - {\rm j})\cdot \sqrt{\frac {R\hspace{0.03cm}' }{ 2 \cdot f \cdot 2 \pi | C\hspace{0.03cm}'}}= (1 - {\rm j})\cdot \sqrt{\frac {R\hspace{0.03cm}' }{ 2 \cdot f \cdot 2 \pi | ||
C\hspace{0.03cm}'}}\hspace{0.05cm}.$$ | C\hspace{0.03cm}'}}\hspace{0.05cm}.$$ | ||
| − | Mit den angegebenen Leitungsbeschlägen erhält man: | + | *Mit den angegebenen Leitungsbeschlägen erhält man: |
:$${\rm Re}\{Z_{\rm W}(f= 1\,{\rm kHz})\} = \sqrt{\frac {100\,{\rm \Omega/km }}{ 2 \cdot 10^{3}\,{\rm Hz} \cdot 2 \cdot 10^{-7} | :$${\rm Re}\{Z_{\rm W}(f= 1\,{\rm kHz})\} = \sqrt{\frac {100\,{\rm \Omega/km }}{ 2 \cdot 10^{3}\,{\rm Hz} \cdot 2 \cdot 10^{-7} | ||
\,{\rm s/(\Omega \cdot km) }}} \hspace{0.15cm}\underline{= 500\,{\rm | \,{\rm s/(\Omega \cdot km) }}} \hspace{0.15cm}\underline{= 500\,{\rm | ||
Version vom 6. November 2019, 15:16 Uhr
Wir gehen von einer homogenen und reflektionsfrei abgeschlossenen Leitung der Länge $l$ aus, so dass für die Spektralfunktion am Ausgang gilt:
- $$U_2(f) = U_1(f) \cdot {\rm e}^{-\hspace{0.02cm}\gamma(f) \hspace{0.05cm} \cdot \hspace{0.05cm}l} \hspace{0.05cm}.$$
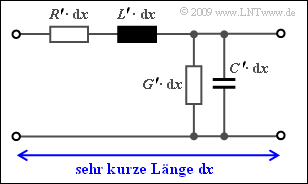
Hierbei beschreibt $\gamma(f)$ das Übertragungsmaß einer extrem kurzen Leitung der infinitesimalen Länge $dx$, das man mit den Belägen $R\hspace{0.05cm}'$, $L\hspace{0.05cm}'$, $G\hspace{0.08cm}'$ und $C\hspace{0.08cm}'$ (siehe Grafik) wie folgt darstellen kann:
- $$\gamma(f) = \sqrt{(R\hspace{0.05cm}' + {\rm j} \cdot 2\pi f \cdot L\hspace{0.05cm}') \cdot (G\hspace{0.08cm}' + {\rm j} \cdot 2\pi f \cdot C\hspace{0.08cm}')} = \alpha (f) + {\rm j} \cdot \beta (f)\hspace{0.05cm}.$$
Der Realteil von $\gamma(f)$ ergibt
- das Dämpfungsmaß $\alpha(f)$ , und
- der Imaginärteil das Phasenmaß $\beta(f)$.
Nach einiger Rechnung kann man für diese Größen schreiben:
- $$\alpha(f) = \sqrt{{1}/{2}\cdot \left (R\hspace{0.05cm}' \cdot G\hspace{0.08cm}' - \omega^2 \cdot L\hspace{0.05cm}' \cdot C\hspace{0.08cm}'\right)+ {1}/{2}\cdot \sqrt{(R\hspace{0.05cm}'\hspace{0.05cm}^2 + \omega^2 \cdot L\hspace{0.05cm}'\hspace{0.05cm}^2) \cdot (G\hspace{0.08cm}'\hspace{0.05cm}^2 + \omega^2 \cdot C\hspace{0.08cm}'\hspace{0.05cm}^2)}} \hspace{0.1cm}\bigg |_{\hspace{0.05cm}\omega \hspace{0.05cm}= \hspace{0.05cm}2\pi f},$$
- $$\beta(f) = \sqrt{{1}/{2}\cdot \left (-R\hspace{0.05cm}' \cdot G\hspace{0.08cm}' + \omega^2 \cdot L\hspace{0.05cm}' C\hspace{0.08cm}'\right)+ {1}/{2}\cdot \sqrt{(R\hspace{0.05cm}'\hspace{0.05cm}^2 + \omega^2 \cdot L\hspace{0.05cm}'\hspace{0.05cm}^2) \cdot (G\hspace{0.08cm}'\hspace{0.05cm}^2 + \omega^2 \cdot C\hspace{0.08cm}'\hspace{0.05cm}^2)}} \hspace{0.1cm}\bigg |_{\hspace{0.05cm}\omega \hspace{0.05cm}= \hspace{0.05cm}2\pi f}.$$
Bei der Dämpfungsfunktion $a(f)$ ist zusätzlich die Pseudoeinheit „Neper” (Np) hinzuzufügen und bei der Phasenfunktion $b(f)$ „Radian” (rad). Da die Leitungsbeläge jeweils auf die Leitungslänge bezogen sind, weisen $\alpha(f)$ bzw. $\beta(f)$ die Einheiten „Np/km” bzw. „rad/km” auf.
Eine weitere wichtige Beschreibungsgröße neben $\gamma(f)$ ist der Wellenwiderstand $Z_{\rm W}(f)$, der an jedem Ort den Zusammenhang zwischen Spannung und Strom der beiden laufenden Wellen angibt. Es gilt:
- $$Z_{\rm W}(f) = \sqrt{\frac {R\hspace{0.05cm}' + {\rm j} \cdot \omega L\hspace{0.05cm}'}{G\hspace{0.08cm}' + {\rm j} \cdot \omega C\hspace{0.08cm}'}} \hspace{0.1cm}\bigg |_{\hspace{0.05cm} \omega \hspace{0.05cm}= \hspace{0.05cm}2\pi f}.$$
Hinweise:
- Die Aufgabe gehört zum Kapitel Einige Ergebnisse der Leitungstheorie.
- Verwenden Sie für die numerischen Berechnungen jeweils die Zahlenwerte
- $$R\hspace{0.05cm}' = 100\,\,{\rm \Omega}/{ {\rm km} }\hspace{0.05cm},\hspace{0.3cm} G\hspace{0.08cm}' = 1\,\,{\rm µ S}/{ {\rm km}}\hspace{0.05cm},\hspace{0.3cm} 2\pi L\hspace{0.03cm}' = 2\,\,{\rm mH}/{ {\rm km}} \hspace{0.05cm},\hspace{0.3cm} 2\pi C\hspace{0.08cm}' = 200\,\,{\rm nF}/{ {\rm km}} \hspace{0.05cm}.$$
Fragebogen
Musterlösung
- $$\alpha(f = 0) = [1\,{\rm Np}] \hspace{-0.03cm}\cdot \hspace{-0.03cm} \sqrt{{1}/{2}\hspace{-0.03cm}\cdot \hspace{-0.03cm} R\hspace{0.03cm}' \hspace{-0.03cm}\cdot \hspace{-0.03cm} G\hspace{0.03cm}'+ {1}/{2}\cdot R\hspace{0.03cm}' \hspace{-0.03cm}\cdot \hspace{-0.03cm} G\hspace{0.03cm}'} = [1\,{\rm Np}] \hspace{-0.03cm}\cdot \hspace{-0.03cm} \sqrt{ R\hspace{0.03cm}' \hspace{-0.03cm}\cdot \hspace{-0.03cm} G\hspace{0.03cm}'} = [1\,{\rm Np}] \hspace{-0.03cm}\cdot \hspace{-0.03cm} \sqrt{ 100\,{\rm \Omega/km} \hspace{-0.03cm}\cdot \hspace{-0.03cm} 10^{-6}\,{\rm (\Omega \hspace{-0.03cm}\cdot \hspace{-0.03cm} km})^{-1}} \hspace{0.15cm}\underline{= 0.01\,{\rm Np}/{ {\rm km}} }\hspace{0.05cm},$$
- $$\beta(f = 0) = [1\,{\rm rad}] \cdot \sqrt{-{1}/{2}\cdot R\hspace{0.03cm}' \cdot G\hspace{0.03cm}'+ {1}/{2}\cdot R\hspace{0.03cm}' \cdot G\hspace{0.03cm}'} \hspace{0.15cm}\underline{= 0 }\hspace{0.05cm},$$
- $$Z_{\rm W}(f = 0) = \sqrt{\frac {R\hspace{0.03cm}'}{G\hspace{0.03cm}'}} = \sqrt{\frac {100\,{\rm \Omega/km}}{{\rm 10^{-6}/(\Omega \cdot km})}}\hspace{0.15cm}\underline{= 10\, {\rm k \Omega}}\hspace{0.05cm}.$$
Die Gleichsignaldämpfung wird relevant,
- wenn das Nutzsignal im Basisband übertragen werden soll und einen Gleichanteil besitzt, oder
- wenn der Netzabschluss beim Teilnehmer von der Ortsvermittlungsstelle aus mit Leistung versorgt werden muss (Fernspeisung).
(2) Mit $f = 10^{5} \ \rm Hz$ und den angegebenen Werten gilt
- $$f \cdot 2\pi L' = 10^5\,\frac{1}{ {\rm s}} \cdot 2 \cdot 10^{-3}\,\frac{\rm \Omega \cdot s}{ {\rm km}}= 200 \,\frac{\rm \Omega }{ {\rm km}} \hspace{0.05cm},\hspace{1.05cm} f \cdot 2\pi C' = 10^5\,\frac{1}{ {\rm s}} \cdot 2 \cdot 10^{-7}\,\frac{\rm s}{ {\rm \Omega \cdot km}}= 0.02 \,\frac{\rm 1 }{ {\rm \Omega \cdot km}} \hspace{0.05cm}.$$
Damit ergibt sich für das Dämpfungsmaß in „Np/km”:
- $$\alpha(f = 100\,{\rm kHz}) = \sqrt{ {1}/{2}\cdot \left (100 \cdot 10^{-6} - 200 \cdot 0.02 \right)+ {1}/{2} \cdot \sqrt{(100^2 + 200^2) \cdot (10^{-12} + 0.02^2)}} $$
- $$ \Rightarrow \; \; \alpha(f = 100\,{\rm kHz}) \approx \sqrt{{1}/{2}\cdot \left (10^{-4} - 4 \right)+ {1}/{2}\cdot \sqrt{5 \cdot 10^{4} \cdot 4 \cdot 10^{-4}}} \approx \sqrt {-2 + \frac{\sqrt{20}}{ 2}} \hspace{0.15cm}\underline{\approx 0.486 \ {\rm Np/km}} \hspace{0.05cm}.$$
(3) Der Grenzübergang für $f → \infty$ ergibt sich, wenn man im Zähler $R\hspace{0.03cm}'$ und im Nenner $G\hspace{0.08cm}'$ gegenüber den jeweils zweiten Term vernachlässigt:
- $$\lim_{f \rightarrow \infty} \hspace{0.1cm} Z_{\rm W}(f) = \lim_{\omega \rightarrow \infty} \hspace{0.1cm} \sqrt{\frac {R\hspace{0.03cm}' + {\rm j} \cdot \omega L'}{G' + {\rm j} \cdot \omega C\hspace{0.03cm}'}} =\sqrt{\frac {2 \pi L\hspace{0.03cm}' }{2 \pi C\hspace{0.03cm}'}}=\sqrt{\frac {2 \cdot 10^{-3}\,{\rm \Omega \cdot s} } {2 \cdot 10^{-73}\,{\rm s/\Omega} }} \hspace{0.15cm}\underline{= 100\,{\rm \Omega }}\hspace{0.05cm}.$$
- Die Näherung für die Dämpfungsfunktion ist schwieriger herzuleiten. Ausgehend von
- $$\alpha(\omega) = \sqrt{ {1}/{2}\cdot \left (R\hspace{0.03cm}' G\hspace{0.03cm}' - \omega^2 \cdot L\hspace{0.03cm}' C\hspace{0.03cm}'\right)+ {1}/{2}\sqrt{(R\hspace{0.03cm}'\hspace{0.03cm}^2 + \omega^2 \cdot L\hspace{0.03cm}'\hspace{0.03cm}^2) \cdot (G\hspace{0.03cm}'\hspace{0.03cm}^2 + \omega^2 \cdot C\hspace{0.03cm}\hspace{0.03cm}'^2)}}$$
- gilt dann ebenfalls:
- $$2 \cdot \alpha^2(\omega) = R\hspace{0.03cm}' G\hspace{0.03cm}' + \omega^2 \cdot L' C'\cdot \left [-1 +\sqrt{(1 + \frac{R\hspace{0.03cm}'^2}{ \omega^2 \cdot L\hspace{0.03cm}'^2}) \cdot (1 + \frac{G\hspace{0.03cm}'^2}{ \omega^2 \cdot C\hspace{0.03cm}'^2})} \hspace{0.1cm} \right]$$
- $$\Rightarrow \; \; 2 \cdot \alpha^2(\omega) \approx R\hspace{0.03cm}' G\hspace{0.03cm}' + \omega^2 \cdot L' C\hspace{0.03cm}'\cdot \left [-1 +\sqrt{1 + \frac{R'^2}{ \omega^2 \cdot L'^2}+ \frac{G\hspace{0.03cm}'^2}{ \omega^2 \cdot C\hspace{0.03cm}'^2}} \hspace{0.1cm} \right].$$
- Über die für kleine $x$ gültige Näherung $\sqrt{1 + x}\approx 1+x/2$ kommt man zum Zwischenergebnis für (unendlich) große Frequenzen:
- $$2 \cdot \alpha^2(\omega \rightarrow \infty) = R\hspace{0.03cm}' G\hspace{0.05cm}' + \omega^2 \cdot L' C\hspace{0.05cm}'\cdot \left [ -1 +1 + {1}/{2} \cdot \left ( \frac{R\hspace{0.03cm}'\hspace{0.03cm}^2}{ \omega^2 \cdot L\hspace{0.03cm}'\hspace{0.03cm}^2}+ \frac{G\hspace{0.03cm}'\hspace{0.03cm}^2}{ \omega^2 \cdot C\hspace{0.03cm}'\hspace{0.03cm}^2} \right) \hspace{0.1cm} \right] $$
- $$\Rightarrow \hspace{0.3cm} 2 \cdot \alpha^2(\omega \rightarrow \infty) = \frac{2 \cdot R\hspace{0.03cm}' G\hspace{0.03cm}' C\hspace{0.03cm}' L'+ R\hspace{0.03cm}'\hspace{0.03cm}^2 C\hspace{0.03cm}'\hspace{0.03cm}^2+ G\hspace{0.03cm}'\hspace{0.03cm}^2 L\hspace{0.03cm}'\hspace{0.03cm}^2}{2 \cdot C\hspace{0.03cm}' L\hspace{0.03cm}' }= \frac{(R\hspace{0.03cm}' C\hspace{0.03cm}' + G\hspace{0.03cm}' L\hspace{0.03cm}')^2}{2 \cdot C\hspace{0.03cm}' L\hspace{0.03cm}' }$$
- $$\Rightarrow \hspace{0.3cm} \alpha(\omega \rightarrow \infty) = {1}/{2}\cdot \frac{R\hspace{0.03cm}' C\hspace{0.03cm}' + G\hspace{0.03cm}' L\hspace{0.03cm}'}{\sqrt{ C\hspace{0.03cm}' L\hspace{0.03cm}' }}= {1}/{2}\cdot \left [R\hspace{0.03cm}' \cdot \sqrt{\frac{C\hspace{0.03cm}'}{L\hspace{0.03cm}'}}+G\hspace{0.03cm}' \cdot \sqrt{\frac{L\hspace{0.03cm}'}{C\hspace{0.03cm}'}}\right]\hspace{0.05cm}.$$
- Mit den eingesetzten Zahlenwerten ergibt sich
- $$\alpha(f \rightarrow \infty) = \alpha(\omega \rightarrow \infty) = {0.5\,{\rm Np/km}}\cdot \left [100 \cdot \sqrt{\frac{2 \cdot 10^{-7}}{2 \cdot10^{-3}}}+10^{-6} \cdot \sqrt{\frac{2 \cdot10^{-3}}{2 \cdot10^{-7}}}\right] \hspace{0.15cm}\underline{\approx 0.5 \, {\rm Np}/{\rm km}}\hspace{0.05cm}.$$
(4) Für kleine Frequenzen gilt $\omega L\hspace{0.03cm}' \ll R\hspace{0.03cm}'$ und $ \omega C\hspace{0.03cm}' \gg G\hspace{0.03cm}'$.
- Unter Vernachlässigung des $\omega^2$–Anteils erhält man:
- $$\alpha(f) = \sqrt{\frac {1}{2}\cdot \left (R\hspace{0.03cm}' G\hspace{0.03cm}' - \omega^2 \cdot L\hspace{0.03cm}' C\hspace{0.03cm}'\right)+ \frac {1}{2}\sqrt{(R\hspace{0.03cm}'\hspace{0.03cm}^2 + \omega^2 \cdot L\hspace{0.03cm}'\hspace{0.03cm}^2) \cdot (G\hspace{0.03cm}'\hspace{0.03cm}^2+ \omega^2 \cdot C\hspace{0.03cm}'\hspace{0.03cm}^2)}} \hspace{0.1cm}\bigg |_{\hspace{0.05cm}\omega \hspace{0.05cm}= \hspace{0.05cm}2\pi f}$$
- $$ \Rightarrow \hspace{0.3cm} \alpha(f) \approx \sqrt{\frac {R\hspace{0.03cm}' G\hspace{0.03cm}'}{2}+ \frac {R\hspace{0.03cm}' \cdot \omega C\hspace{0.03cm}'}{2}} \hspace{0.1cm}\bigg |_{\hspace{0.03cm}\omega \hspace{0.05cm}= \hspace{0.05cm}2\pi f} \approx \sqrt{ {1}/{2} \cdot f \cdot R\hspace{0.03cm}' \cdot 2 \pi C\hspace{0.03cm}'} \hspace{0.05cm}.$$
- Hierbei ist berücksichtigt, dass der erste Anteil gemäß Teilaufgabe (1) außer bei der Frequenz $f = 0$ vernachlässigt werden kann.
- Für die Frequenz $f = 1 \ \rm kHz$ ergibt sich die Näherung
- $$\alpha(f = 1\,{\rm kHz}) = \sqrt{ {1}/{2} \cdot 10^{3}\,{\rm Hz} \cdot 100\,\frac{\rm \Omega }{ {\rm km}} \cdot 2 \cdot 10^{-7} \,\frac{\rm s }{ {\rm \Omega \cdot km}}} \hspace{0.15cm}\underline{= 0.1\,{\rm Np }/{ {\rm km}}} \hspace{0.05cm}.$$
- Für die Frequenz $f = 4 \ \rm kHz$ ist das Dämpfungsmaß doppelt so groß:
- $$\alpha(f = 4\,{\rm kHz}) \hspace{0.15cm}\underline{ = 0.2\,{\rm Np }/{ {\rm km}}} \hspace{0.05cm}.$$
(5) Für den Wellenwiderstand gilt bei niedrigen Frequenzen näherungsweise:
- $$Z_{\rm W}(f) = \sqrt{\frac {R\hspace{0.03cm}' + {\rm j} \cdot f \cdot 2 \pi L\hspace{0.03cm}'}{G\hspace{0.03cm}' + {\rm j} \cdot f \cdot 2 \pi C\hspace{0.03cm}'}} \approx \sqrt\frac{1 }{ {\rm j}} \cdot \sqrt{\frac {R\hspace{0.03cm}' }{ f \cdot 2 \pi C\hspace{0.03cm}'}}= (1 - {\rm j})\cdot \sqrt{\frac {R\hspace{0.03cm}' }{ 2 \cdot f \cdot 2 \pi C\hspace{0.03cm}'}}\hspace{0.05cm}.$$
- Mit den angegebenen Leitungsbeschlägen erhält man:
- $${\rm Re}\{Z_{\rm W}(f= 1\,{\rm kHz})\} = \sqrt{\frac {100\,{\rm \Omega/km }}{ 2 \cdot 10^{3}\,{\rm Hz} \cdot 2 \cdot 10^{-7} \,{\rm s/(\Omega \cdot km) }}} \hspace{0.15cm}\underline{= 500\,{\rm \Omega}}\hspace{0.05cm},$$
- $$ {\rm Im}\{Z_{\rm W}(f= 1\,{\rm kHz})\} = -{\rm Re}\{Z_{\rm W}(f= 1\,{\rm kHz})\}\hspace{0.15cm}\underline{= -500\,{\rm \Omega}}\hspace{0.05cm}.$$